Fractaled Atlas

By Maryam Zaringhalam, @thisisartlab

No matter where we look in the natural world, we are sure to find recurring patterns. As a result, natural scientists devote their careers to [humbly] attempt to find and define these very patterns. The most abundant of these natural motifs is arguably the fractal—a geometric structure that can be subdivided into smaller parts that look roughly similar to the whole. Take the branching pattern of the veins on a leaf as an example: zoom into one of those branches, and you’ll find that it’s reminiscent of the overarching branching structure // zoom into one of those branches’ branches and you’ll find the same thing… over and over again!

At their core, fractals are simply the geometric result of repeating the same pattern over and over at a smaller and smaller scale—increasingly tiny patterns within a greater overarching motif. But fractals are the ultimate paradox. Though they are built on simple repetitions, they are infinitely complex. You can subdivide // zoom in // subdivide // zoom in and you’ll still see the same [or similar] patterns emerging and repeating with detail at all scales. Nature is built on these repetitions, all the way down to the subatomic level—the quarks // leptons // bosons.1

“Philosophy is … written in the language of mathematics, and its characters are triangles, circles, and other geometric figures … without these, one is wandering about in a dark labyrinth.” —Galileo Galilei

The ultimate quest for a mathematician is to define simple equations with far-reaching consequences: a2 + b2 = c2 // eiπ + 1 = 0 // E=mc2. The aim is to distill limitless complexity down into elegant and powerful formulas. Surprisingly, despite the natural abundance of the fractal form, it was not until the 20th century that mathematicians even really began investigating fractal structures and their geometry. In fact, it was not even until 1975 that mathematician Benoît Mandelbrot even gave a name to these forms! Mandelbrot is largely credited with elevating the study of fractals to its prominence today, beginning with his discovery of the Mandelbrot set.2

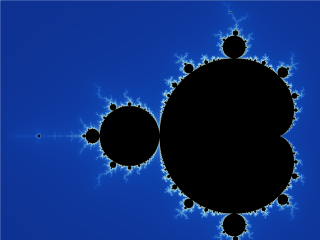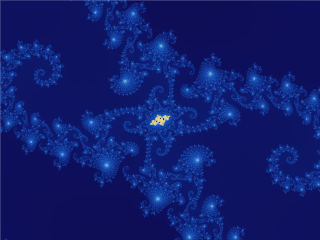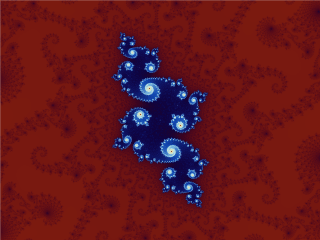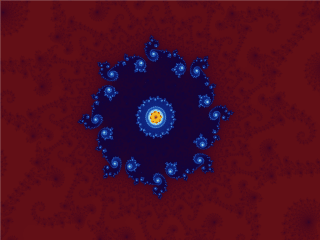
Mandelbrot created his revolutionary // revelationary set essentially by assigning every point on a screen with a unique number. He then plugged each number into a formula, got a result, and plugged the result back into the formula.3 Over and over. Millions and billions of times. In this manner, Mandelbrot tracked the fate of each point on the screen; either it grew to infinity or shrank to zero through these repetitions. If the initial number shrank—was bounded— he colored the point black. If, however, it grew—or escaped—to infinity, he assigned the point a particular color based on how many times he could repeat the formula before the result became exceedingly large.

“I never had the feeling that my imagination was rich enough to invent all those extraordinary things … They were there, even though nobody had seen them before. It’s marvelous … the goal of science is starting with a mess, and explaining it with a simple formula, a kind of dream of science.” —Benoît Mandelbrot

Point by point, Mandelbrot transformed a mathematical repetition into a visual, fractal form. Amazingly, you can zoom in and in on the result and find limitless repetitions of the same basic pattern so that there is complexity at all levels—infinite resolution.

The Mandelbrot set is often called “the thumbprint of God” not only because it is a visual representation of the infinite + eternal, but also because it is the mathematical embodiment of so many [if not all] of nature’s forms. [Fingerprints are also yet another great example of fractals!] If beauty really is in the details, then the Mandelbrot set—and fractals more broadly—provides an infinite source of beauty.

With the rise of computing capabilities, fractal art has gained a major foothold in the art world, as artists have begun applying this mathematical elegance to their work. Electric Sheep, the brainchild of Scott Draves, is an iterative screensaver that is continually evolving based on the aesthetic selective pressures of over 450,000 participants: “It’s all about how pattern emerges from chaos and random stuff.”

The project has taken fractal art to the next level by incorporating animation into the fractal framework to generate abstract animations using the fractal Flame algorithm. This algorithm treats each pixel on a screen like a particle and moves it based on an equation that is reiterated. The animations are the result of the interactions of billions of interweaving pixels that move according to the algorithm’s instructions. These animations are then collectively ranked by participants using the Electric Sheep screen savers, and the most highly ranked—the most fit—mate to produce new animations for the next generation [an iterative process of evolution by aesthetic selection].

Given that the algorithms that code for the emergence of the Electric Sheep are based in natural forms and phenomena, it is no surprise that our computers’ dreams flow organically into and out of each other, endlessly looping into new and beautiful forms. For me, Electric Sheep is a prime example of the art inherent in these most fundamental models for the natural world. Of how seemingly abstract numbers and figures have the potential to inform and add a new dimension to art.

This post was originally featured on Maryam’s blog, ArtLab. To read more of her thoughts on the intersection of art and science, check out http://thisisartlab.com.

________________________________________________

1 Unlike mathematically generated fractals like the Mandelbrot set, fractals found in nature are not infinitely repetitive. The repetition ends at the subatomic level because, at least to our current knowledge, there is no unit smaller than a subatomic particle.

2 The Mandelbrot set was first published in Scientific American for a broad audience in 1985: “Computer Recreations: A computer microscope zooms in for a look at the most complex object in mathematics.”

3 If you’re interested in some more mathematical detail, he called each unique point c. He then plugged each value for c into the formula f(z) = z2 + c using z = 0 and calculated what number came out. He took the result, plugged it back in as the new z value and saw what number came out again. The output of the equation becomes the input for the next iteration of that operation. This mathematical process is called recursion.